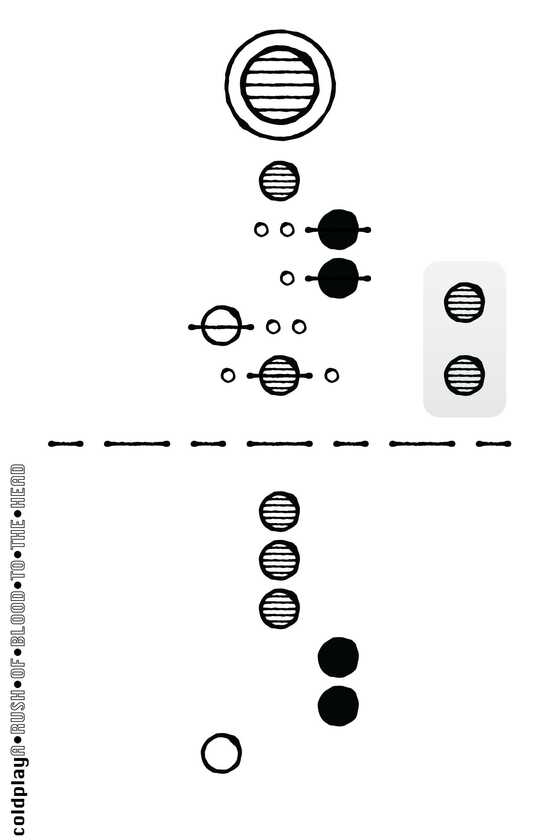
Music is a language. A language that your ears naturally understand, but that’s also utterly foreign to your eyes … as a mess of confusing dots and lines and squiggles.

It’s frustrating, to say the least. Because it means that you’re already fluent in this language (or at least you can easily hear it), but at the same time you’re not able to speak it (by easily playing and creating it yourself).
And as long as you’re confused by the visual language of music, you’ll struggle with the syntax of song, unable to fully articulate the music that you can hear inside.
So what’s needed is a way to align your hearing with your sight. That is, you need a method to illustrate the patterns of music in such a way that they LOOK as natural and simple as they SOUND.
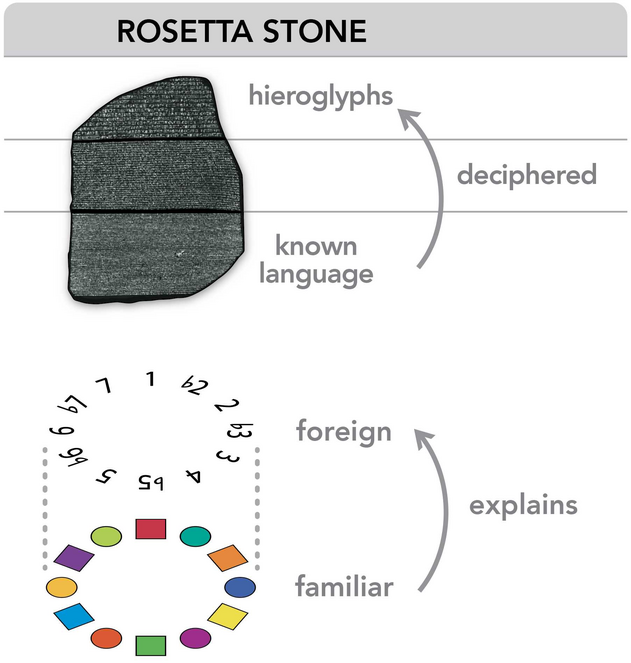
In other words, you need a “Rosetta Stone” to help bridge the gap between the musical language of your ears and that of your eyes.
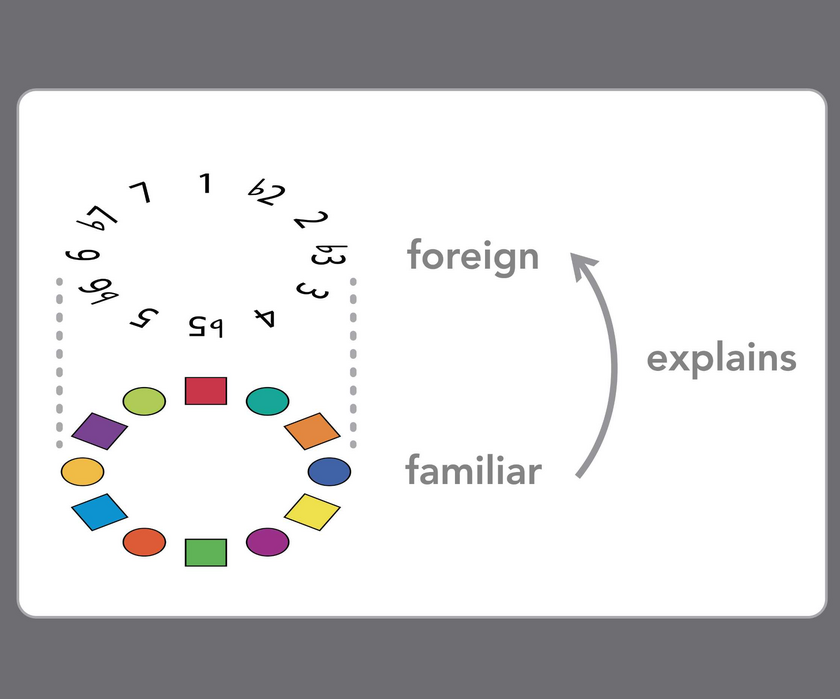
And with color, you have the answer — using the special connection between the circle of fifths and the color wheel, along with the natural link between the circle of fifths and the chromatic scale.
By leveraging your familiarity with color to decipher the otherwise cryptic sequence notes, you can finally crack the code of music. This is our musical Rosetta Stone.